Cartesian equation of a line

A line is a one-dimensional figure that only has length and no width. In this article, we will learn about the equation of a line on a cartesian plane.

If you have ever played video games, you may not know it, but under the hood is a heap of 3D geometry being used to give you the best experience. Just one example of this is ray tracing: a method of simulating light within video games to make it seem natural. Ray tracing is done by modelling lots of rays of light coming in a straight line from the light source, and seeing which targets they hit. This allows the positions of shadows and reflections to be calculated accurately, to make an immersive, realistic-looking world. This is just one example of how 3D geometry, and in particular 3D lines, are used in everyday life. Explore our app and discover over 50 million learning materials for free.

Cartesian equation of a line

Vectors can be defined as a quantity possessing both direction and magnitude. Position vectors simply denote the position or location of a point in the three-dimensional Cartesian system with respect to a reference origin. Further, we shall study in detail about vectors and Cartesian equation of a line in three dimensions. It is known that we can uniquely determine a line if:. Let us study each case separately and try to determine the equation of a line in both the given cases. Therefore, the vector equation of a line passing through a given point and parallel to a given vector is given by:. Substituting these values in the vector equation of a line passing through a given point and parallel to a given vector and equating the coefficients of unit vectors i, j and k, we have,. Substituting these values in the vector equation of a line passing through two given points and equating the coefficients of unit vectors i, j and k, we have. Your Mobile number and Email id will not be published. Post My Comment. Maths Math Article Equation Line. Did not receive OTP? Share Share Share Call Us.

Vectors can be added and subtracted by adding or subtracting the individual components. Maths Math Article Equation Line. For a vector in unit vector form, this just means expanding the brackets in the usual way.

The cartesian form helps to represent geometric entities in the cartesian plane. A point, a line, or a plane can be easily represented in a three-dimensional plane, across the x-axis, y-axis, z-axis, in cartesian form. The cartesian form is helpful to represent the geometric entities as algebraic expressions in three-dimensional geometry. Let us learn more about the conversion of cartesian form to vector form, the difference between cartesian form and vector form, with the help of examples, FAQs. The cartesian form helps in representing a point, a line, or a plane in a two-dimensional or a three-dimensional plane.

The cartesian form helps to represent geometric entities in the cartesian plane. A point, a line, or a plane can be easily represented in a three-dimensional plane, across the x-axis, y-axis, z-axis, in cartesian form. The cartesian form is helpful to represent the geometric entities as algebraic expressions in three-dimensional geometry. Let us learn more about the conversion of cartesian form to vector form, the difference between cartesian form and vector form, with the help of examples, FAQs. The cartesian form helps in representing a point, a line, or a plane in a two-dimensional or a three-dimensional plane. The cartesian form is represented with respect to the three-dimensional cartesian system and is with reference to the x-axis, y-axis, and z-axis respectively. The representation of a point in a three-dimensional cartesian plane is x, y, z , and each of x,y, z represent the coordinates of the points with respect to the x-axis, y-axis, and z-axis respectively. The cartesian form can be easily transformed into vector form, and the same vector form can be transformed back to cartesian form. This can be done using two simple techniques. Secondly, the formula of the product of unit vectors is helpful in converting the cartesian form to vector form.

Cartesian equation of a line

Forgot password? New user? Sign up. Existing user? Log in. Already have an account? Log in here. To find the equation of a line in a two-dimensional plane, we need to know a point that the line passes through as well as the slope.

Evozi apps

Let's see some examples, where you must determine whether lines are skewed, parallel, or intersecting. What is parametric equation of line in 3D? If it is not true, the lines do not intersect. Read full. This can be represented in the above vector form to obtain the required cartesian form of equation of a line. Help your child perfect it through real-world application. We can represent the equation of the line in vector form. You have to decide on which two equations you are going to solve first. The x-axis, y-axis, and z-axis represent the equation of a line on a cartesian plane. Two lines are skew if they are not parallel and do not intersect. Determine whether the following lines intersect. Above is an example of skewed lines. Save Article.

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Search for courses, skills, and videos.

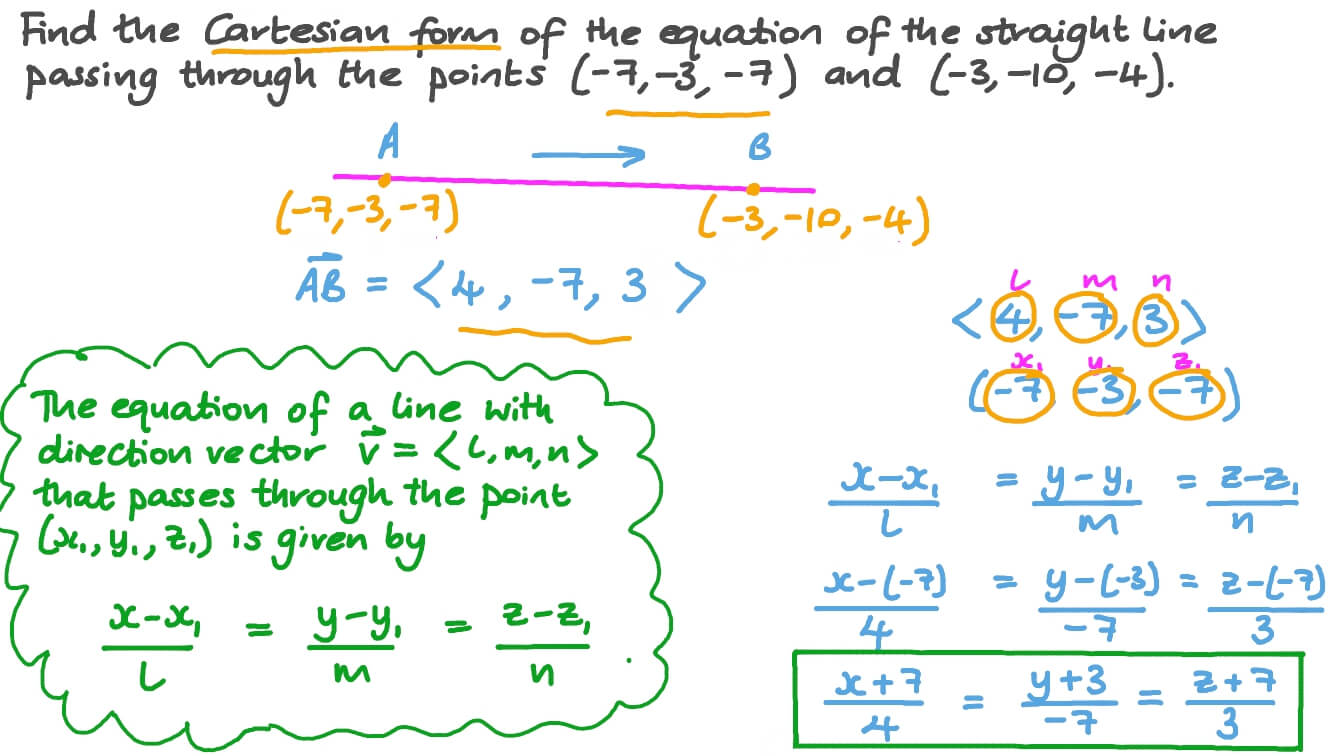
The required equation of the line in cartesian form is as follows. The following points presents the difference between the cartesian form and vector form. In 3D, there is no exact "slope" of a straight line, so instead the direction vector determines which direction the line is going. Inverse Sine. United States. For a vector in unit vector form, this just means expanding the brackets in the usual way. Practice Questions 6. Two lines are skew if they are not parallel and do not intersect. In this study material notes, we learned how to find a line equation on a cartesian plane. Secondly, the formula of the product of unit vectors is helpful in converting the cartesian form to vector form. We can also represent this expression as A x, y, z. Exam Pattern for Class 11th. If it is true, the lines intersect, and if it is false, the lines must be skewed, since you showed that they are not parallel. Find out more details about an inverse function graph here.