Cos2x
The trigonometric ratio of cos2x angle in a right triangle describes the relationship between the angle and the lengths of its sides. One such trigonometric ratio is cos 2X. The cos2x formula is as follows, cos2x.
The subject originally thought and part of the scope of development to solve geometric problems involving triangles. We know about the trigonometric ratios of acute angles as the ratio of the sides of a right-angle triangle. In this Chapter, we will generalize the concept and Cos 2X formula of one such trigonometric ratios namely cos 2X with other trigonometric ratios. Let us start with our learning! The trigonometric ratios of an angle in a right triangle define the relationship between the angle and the length of its sides.
Cos2x
Welcome to another enlightening piece on Brighterly , your most reliable platform for illuminating mathematical concepts. The trigonometric function Cos2x. From the swinging of a pendulum to the orbits of planets, the principles encapsulated by Cos2x are integral to our comprehension of the natural world. As daunting as it may seem, breaking it down makes it a lot more approachable. In the wonderful world of trigonometry, the term Cos2x may seem intimidating at first, but fear not, young mathematicians! Unpacking the mysteries of Cos2x begins with understanding what cosine itself is and how we define this particular function. The definition of Cos2x revolves around the concept of trigonometric functions and the geometry of the unit circle. For an angle x, Cos2x represents the cosine of the double of this angle. So, if we have an angle x, we calculate Cos2x by finding the cosine of the angle that is twice as large as x. To put it into practice, imagine we have an angle of 30 degrees. The Cos2x value for this angle would be the cosine of 60 degrees.
Trigonometry formula Percentage formula Simple interest formula Distance formula Standard deviation formula Mean formula Area of a square formula Mode formula, cos2x. Factorizing this quadratic cos2x with variable sin a.
Cos2x is one of the important trigonometric identities used in trigonometry to find the value of the cosine trigonometric function for double angles. It is also called a double angle identity of the cosine function. The identity of cos2x helps in representing the cosine of a compound angle 2x in terms of sine and cosine trigonometric functions, in terms of cosine function only, in terms of sine function only, and in terms of tangent function only. Cos2x identity can be derived using different trigonometric identities. Let us understand the cos2x formula in terms of different trigonometric functions and its derivation in detail in the following sections.
Welcome to another enlightening piece on Brighterly , your most reliable platform for illuminating mathematical concepts. The trigonometric function Cos2x. From the swinging of a pendulum to the orbits of planets, the principles encapsulated by Cos2x are integral to our comprehension of the natural world. As daunting as it may seem, breaking it down makes it a lot more approachable. In the wonderful world of trigonometry, the term Cos2x may seem intimidating at first, but fear not, young mathematicians! Unpacking the mysteries of Cos2x begins with understanding what cosine itself is and how we define this particular function. The definition of Cos2x revolves around the concept of trigonometric functions and the geometry of the unit circle. For an angle x, Cos2x represents the cosine of the double of this angle. So, if we have an angle x, we calculate Cos2x by finding the cosine of the angle that is twice as large as x. To put it into practice, imagine we have an angle of 30 degrees.
Cos2x
In a right triangle, the trigonometric ratio of an angle explains the relationship that exists between the angle and the length of its sides. But then what is cos 2x? Cosine 2x or Cos 2x formula is also one such trigonometric formula, which is also known as double angle formula. It is called a double angle formula because it has a double angle in it. This is the reason why it is driven by the expressions for trigonometric functions of the sum and difference of two numbers angles and related expressions. Now that we know what the cos 2x formula is, we can move ahead and learn some more important things about trigonometry and also know what the formula of cos2x is. In a right-angled triangle, the hypotenuse, the base adjacent , and the perpendicular opposite , i.
Twitch bit scam listesi
Customize your course in 30 seconds Which class are you in? Test Series. The definition of Cos2x revolves around the concept of trigonometric functions and the geometry of the unit circle. The formula for cos 2x is derived by using the sum angle formula of cosine function. These formulas are utilised to simplify complex trigonometric expressions and solve integration problems. Example 1: Prove the triple angle identity of cosine function using cos2x formula. Trigonometry formula Percentage formula Simple interest formula Distance formula Standard deviation formula Mean formula Area of a square formula Mode formula. To derive the cos2x formula in terms of tan x, we will employ a few trigonometric identities and trigonometric formulas such as. Beyond teaching, I am passionate about spending time with my family, reading, and watching movies. Welcome onboard. The formula for 1 cos 2 x is derived from trigonometric identities. For any given angle x, Cos2x is the cosine of the angle that is twice as large as x. Let us start with our learning! Select your account.
Cos2x is one of the important trigonometric identities used in trigonometry to find the value of the cosine trigonometric function for double angles. It is also called a double angle identity of the cosine function. The identity of cos2x helps in representing the cosine of a compound angle 2x in terms of sine and cosine trigonometric functions, in terms of cosine function only, in terms of sine function only, and in terms of tangent function only.
Cos2x is a double angle trigonometric function that determines the value of cos when the angle x is doubled. Acceleration formula Power formula Velocity formula Average speed formula Pressure formula Momentum formula Work formula Displacement formula. Download as PDF. Cos2x and Sec2x are also intertwined, as sec x is simply the reciprocal of cos x. We will use this to prove the identity for cos2x. Home Maths cos2x formula. Let us start with our learning! The Cos2x Formula can be simply demonstrated using the provided identities. One such trigonometric ratio is cos 2X,. Let us consider an example to understand the application of cos2x formula. To calculate the value of cosine double angle,we put the value of angle P and angle O equal to x. This expression provides an alternative way to compute Cos2x when we have the value of sin x but not cos x.

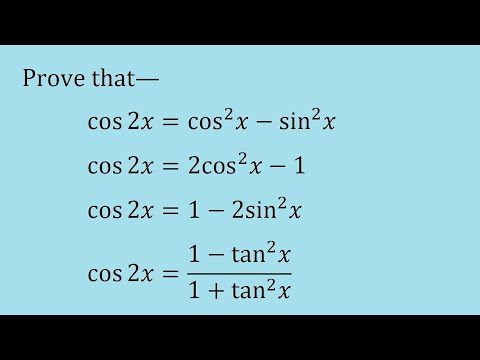
Between us speaking, I would try to solve this problem itself.