Lines of symmetry in a parallelogram

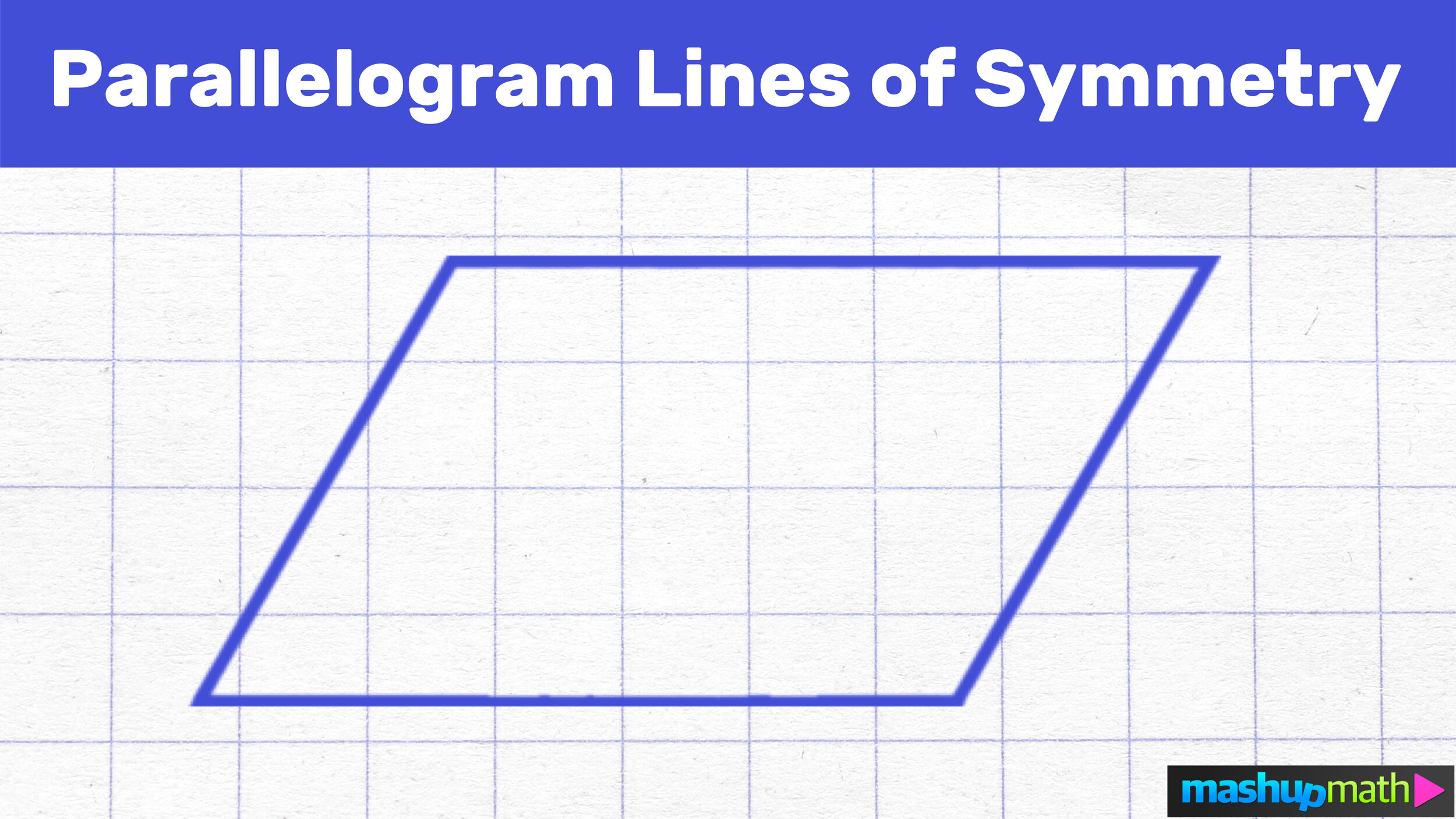
General parallelogram has no lines of symmetry. Some specific types of parallelogram do.

August 12, by Anthony Persico. Every Geometry class or course will include a deep exploration of the properties of parallelograms. In this post, we will quickly review the key properties of parallelograms including their sides, angles, and corresponding relationships. Finally, we will determine whether or not a parallelogram has line symmetry. And, if a parallelogram has line symmetry, what would parallelogram lines of symmetry look like in the form of a diagram. Definition: A parallelogram is a special kind of quadrilateral a closed four-sided figure where opposite sides are parallel to each other and have equal length. Furthermore, the interior opposite angles in any parallelogram have equal value.

Lines of symmetry in a parallelogram

Lines of symmetry in a parallelogram vary from type to type. In simple words, the parallelogram lines of symmetry refer to the lines which cut the parallelogram into two identical parts. To recall, a parallelogram is a quadrilateral 4-sided figure where the opposite sides are parallel to each other. The lines of symmetry are those lines which divide a parallelogram into two halves where each half is the mirror image of the other. Different parallelograms have different lines of symmetry and the different number of symmetry lines. There are three types of a parallelogram whose number of symmetry lines are given in the aforementioned table. Below are the explanations on the lines of symmetry in each of these parallelograms. In a square, there are four lines of symmetry, each of which divides it into two identical parts. The symmetry lines of a square are both its diagonals and the lines joining the midpoints of its opposite sides bisectors. There are two lines of symmetry in a rectangle which cuts it into two equal halves. In a rectangle, the lines of symmetry are those lines which join the midpoint of the opposite and parallel lines i.

In a square, there are four lines of symmetry, each of which divides it into two identical parts.

Below are pictures of four quadrilaterals: a square, a rectangle, a trapezoid and a parallelogram. This task provides students a chance to experiment with reflections of the plane and their impact on specific types of quadrilaterals. It is both interesting and important that these types of quadrilaterals can be distinguished by their lines of symmetry. The only pictures missing here, from this point of view, are those of a rhombus and a general quadrilateral which does not fit into any of the special categories considered here. This task is best suited for instruction although it could be adapted for assessment. If students have not yet learned the terminology for trapezoids and parallelograms, the teacher can begin by explaining the meaning of those terms. The students should try to visualize the lines of symmetry first, and then they can make or be provided with cutouts of the four quadrilaterals or trace them on tracing paper.

Lines of symmetry in a parallelogram vary from type to type. In simple words, the parallelogram lines of symmetry refer to the lines which cut the parallelogram into two identical parts. To recall, a parallelogram is a quadrilateral 4-sided figure where the opposite sides are parallel to each other. The lines of symmetry are those lines which divide a parallelogram into two halves where each half is the mirror image of the other. Different parallelograms have different lines of symmetry and the different number of symmetry lines. There are three types of a parallelogram whose number of symmetry lines are given in the aforementioned table.

Lines of symmetry in a parallelogram

Always on the lookout for fun math games and activities in the classroom? Try our ready-to-go printable packs for students to complete independently or with a partner! Here you will learn about lines of symmetry, including symmetry properties within polygons, angle properties, and symmetry of different line graphs. Students first learn about line symmetry in grade 4 with their work with 2D shapes in geometry. Lines of symmetry are straight lines that divide a shape into two equal parts, where one part is an exact reflection or mirror image of the other. Regular polygons are polygons that have equal side lengths and equal angle measures. Notice how the number of lines of symmetry in these regular polygons is equal to the number of sides and angles the polygons have. All regular polygons share the property that the number of sides is equal to the number of lines of symmetry. Irregular polygons are polygons that do not have all equal angles and all equal side lengths. However, they can have line symmetry.

You got this meme

Rotational symmetry occurs when an object is rotated in a specific direction, specifically around a point. Solution The lines of symmetry for each of the four quadrilaterals are shown below: When a geometric figure is folded about a line of symmetry, the two halves match up so if the students have copies of the quadrilaterals they can test lines of symmetry by folding. Some specific types of parallelogram do. See below. Summary of Parallelogram Lines of Symmetry Let's summarize whatever we have discussed so far in short so that you can prepare your short notes for quick and effective revisions: There are three ways to move geometric shapes around: reflection, rotation, and translation. The lines of symmetry indicated are the only ones for the figures. Multiplying Fractions. Start Quiz. But that's not enough. Ans: A parallelogram has no symmetry lines. Terms and Conditions.

A parallelogram is a type of quadrilateral where the opposite sides are parallel and equal. The imaginary line so formed along which you can fold a figure to obtain the symmetrical halves is referred to as the line of symmetry. Thus, the lines of symmetry of a parallelogram refer to the lines cutting the parallelogram into two identical parts.

So, if the figure retains its exact appearance after it is rotated, around a center point. Mar 5, Rotational symmetry occurs when an object is rotated in a specific direction, specifically around a point. In this article we will discuss the conversion of yards into feet and feets to yard. Or spending way too much time at the gym or playing on my phone. What is the ratio of the area Parallelograms have zero lines of symmetry because it is impossible to draw a line through the center of any parallelogram that divides the figure into two equal halves that are mirror images of each other. Have questions on basic mathematical concepts? It is both interesting and important that these types of quadrilaterals can be distinguished by their lines of symmetry. An imaginary line through which a parallelogram is folded into two halves such that these halves are symmetrical in nature is known as a line of symmetry in a parallelogram or parallelogram lines of symmetry. A line of symmetry is a mathematical reflection that maps any point on the figure back to the figure. Just download it and start your journey toward your dream with Testbook. A zero vector is defined as a line segment coincident with its beginning and ending points. Example 1: What are the angles at which a parallelogram has rotational symmetry? Is a rhombus always a trapezoid?