Root mean square speed

Other sections state that increasing the temperature increases the speeds at which molecules move.

Determine the most probable, average and root-mean-square speed of gas molecules described by the Maxwell-Boltzmann distribution. Maxwell-Boltzmann distribution describes a classical system of distinguishable particles, such as for example molecules. A distribution function for the magnitude of velocity of the molecules is defined as follows. The most probable speed of gas molecules described by the Maxwell-Boltzmann distribution is the speed at which distribution graph reaches its maximum. Thus, if we know the formula of this distribution, we just need to differentiate it and consider the derivative to be equal to zero. Speed for which the derivate equals zero is the most probable speed. The average speed of molecules is the mean of all magnitudes of velocity at which molecules of the given gas are moving.

Root mean square speed

We have examined pressure and temperature based on their macroscopic definitions. Pressure is the force divided by the area on which the force is exerted, and temperature is measured with a thermometer. We can gain a better understanding of pressure and temperature from the kinetic theory of gases , the theory that relates the macroscopic properties of gases to the motion of the molecules they consist of. First, we make two assumptions about molecules in an ideal gas. To derive the ideal gas law and the connection between microscopic quantities such as the energy of a typical molecule and macroscopic quantities such as temperature, we analyze a sample of an ideal gas in a rigid container, about which we make two further assumptions:. The collisions between molecules do not appear in the derivation of the ideal gas law. They do not disturb the derivation either, since collisions between molecules moving with random velocities give new random velocities. Furthermore, if the velocities of gas molecules in a container are initially not random and isotropic, molecular collisions are what make them random and isotropic. We make still further assumptions that simplify the calculations but do not affect the result. First, we let the container be a rectangular box. Second, we begin by considering monatomic gases, those whose molecules consist of single atoms, such as helium. Then, we can assume that the atoms have no energy except their translational kinetic energy; for instance, they have neither rotational nor vibrational energy. Later, we discuss the validity of this assumption for real monatomic gases and dispense with it to consider diatomic and polyatomic gases. These collisions are the source of pressure in a gas. As the number of molecules increases, the number of collisions, and thus the pressure, increases.

Find the absolute temperature using the Celsius to Kelvin conversion formula:. The result is.

For alternating electric current , RMS is equal to the value of the constant direct current that would produce the same power dissipation in a resistive load. The RMS value of a set of values or a continuous-time waveform is the square root of the arithmetic mean of the squares of the values, or the square of the function that defines the continuous waveform. In physics, the RMS current value can also be defined as the "value of the direct current that dissipates the same power in a resistor. The RMS value of a continuous function or signal can be approximated by taking the RMS of a sample consisting of equally spaced observations. Additionally, the RMS value of various waveforms can also be determined without calculus , as shown by Cartwright.

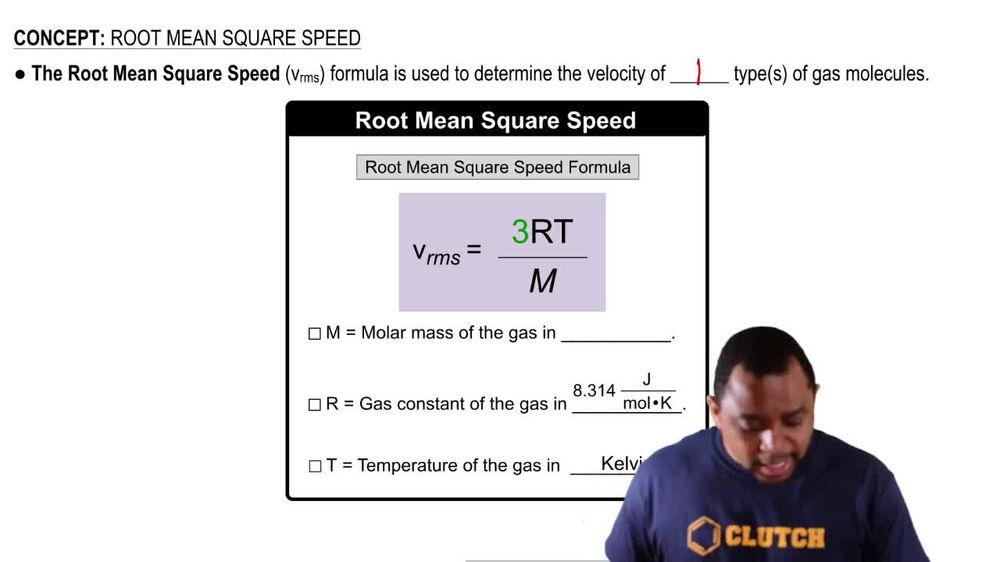
Root mean square speed v rms. Root mean square speed v rms is defined as the square root of the mean of the square of speeds of all molecules. Equation 9. From the equation 9. At a given temperature the molecules of lighter mass move faster on an average than the molecules with heavier masses. We can also write the v rms in terms of gas constant R.

Root mean square speed

For alternating electric current , RMS is equal to the value of the constant direct current that would produce the same power dissipation in a resistive load. The RMS value of a set of values or a continuous-time waveform is the square root of the arithmetic mean of the squares of the values, or the square of the function that defines the continuous waveform. In physics, the RMS current value can also be defined as the "value of the direct current that dissipates the same power in a resistor.

M3gan watch online

The rms velocity is directly proportional to the square root of temperature and inversely proportional to the square root of molar mass. We obtain. We make still further assumptions that simplify the calculations but do not affect the result. The root mean square velocity RMS velocity is a way to find a single velocity value for the particles. First, we make two assumptions about molecules in an ideal gas. Very few helium atoms are left in the atmosphere, but many were present when the atmosphere was formed, and more are always being created by radioactive decay see the chapter on nuclear physics. By taking the square root of both these equations and multiplying them together, the power is found to be:. Use limited data to select advertising. In a mixture of ideal gases in thermal equilibrium, the number of molecules of each gas is proportional to its partial pressure. Further information: Root mean square AC voltage. However, this is not true for an arbitrary waveform, which may not be periodic or continuous. On the other hand, it is much greater than the typical difference in gravitational potential energy when a molecule moves from, say, the top to the bottom of a room, so our neglect of gravitation is justified in typical real-world situations.

The gas laws that we have seen to this point, as well as the ideal gas equation, are empirical, that is, they have been derived from experimental observations. The mathematical forms of these laws closely describe the macroscopic behavior of most gases at pressures less than about 1 or 2 atm. Although the gas laws describe relationships that have been verified by many experiments, they do not tell us why gases follow these relationships.

As we have seen from kinetic theory, when the gases have the same temperature, their molecules have the same average kinetic energy. However, for some purposes the RMS current over a longer period is required when calculating transmission power losses. We digress for a moment to answer a question that may have occurred to you: When we apply the model to atoms instead of theoretical point particles, does rotational kinetic energy change our results? H 2 molecules therefore make 4 times as many collisions with walls. Inception score FID. Gases consist of atoms or molecules that move at different speeds in random directions. Go back to previous article. With the assumption of isotropy, the three averages on the right side are equal, so. Develop and improve services. Kinetic molecular theory tries to explain the properties of gases by investigating the behavior of individual atoms or molecules making up the gas. Answer In a liquid, the molecules are very close together, constantly colliding with one another. The more energy they have, the more room the molecules can make for themselves by expanding against a constant pressure. If the function is periodic such as household AC power , it is still meaningful to discuss the average power dissipated over time, which is calculated by taking the average power dissipation:. Because of their usefulness in carrying out power calculations, listed voltages for power outlets for example, V in the US, or V in Europe are almost always quoted in RMS values, and not peak values.