What is the reciprocal ratio of sine

Trigonometry is all about triangles or to be more precise about the relation between the angles and sides of a right-angled triangle. In this article, what is the reciprocal ratio of sine, we will be discussing about the ratio of sides of a right-angled triangle with respect to its acute angle called trigonometric ratios of the angle and find the reciprocals of these Trigonometric Ratios. The trigonometric ratios of an acute angle in a right triangle are the relationship between the angle and the length of two sides. The ratios defined below are abbreviated as sin C, cos C, and tan C respectively.

The reciprocal of sine is the cosecant function. There are six main trigonometric functions namely, sine, cosine, tangent, cotangent, secant, and cosecant. An important thing to note is that the reciprocal of sine is not the inverse function of sine, that is, the cosecant function is not the inverse function of sine. So, we have cosecant which is the reciprocal of sine. In this article, let us learn about the properties of the reciprocal of sine, that is, cosecant, its formula, domain, range , derivative, integral and graph.

What is the reciprocal ratio of sine

If you're seeing this message, it means we're having trouble loading external resources on our website. To log in and use all the features of Khan Academy, please enable JavaScript in your browser. Search for courses, skills, and videos. The reciprocal trigonometric ratios. About About this video Transcript. Sal finds all six trigonometric ratios sine, cosine, tangent, secant, cosecant, and cotangent of an angle in a given right triangle. Created by Sal Khan. Want to join the conversation? Log in. Sort by: Top Voted. Posted 11 years ago. Direct link to shabarish. What is the full form of csc, sec and cot? Downvote Button navigates to signup page. Flag Button navigates to signup page.

Tarun G Maddila. But I'll define it. Six trigonometric ratios: sine, cosine, tangent, cotangent, secant, and cosecant.

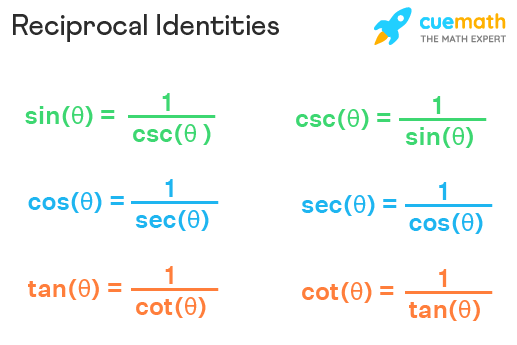
The six trigonometric ratios are sine, cosine, tangent, cotangent, secant, cosecant, out of which the three standard trigonometric ratios are sine, cosine, and tangent. The six trigonometric ratios can be grouped in pairs as reciprocals. The reciprocal identities are the reciprocals of these six trigonometric ratios. Note that reciprocal identities are not the same as inverse trigonometric functions. Reciprocal identities are the reciprocals of the six fundamental trigonometric functions sine, cosine, tangent, secant, cosecant, and cotangent. It is obtained by interchanging the values of numerator and denominator. Similarly, we can find the reciprocal of each trigonometric ratio using their definitions.

The three basic trigonometric functions occur so often as the denominator of a fraction that it is convenient to give names to their reciprocals. We define three new trigonometric functions as follows. We can find exact values for all six trig functions at a given angle if we know the value of any one of them. We can now compute the values of the six trigonometric ratios. By comparing the definitions of secant, cosecant, and cotangent to the three basic trigonometric functions, we find the following relationships. Calculators do not have keys for the secant, cosecant, and cotangent functions; instead, we calculate their values as reciprocals.

What is the reciprocal ratio of sine

The reciprocal of sine is the cosecant function. There are six main trigonometric functions namely, sine, cosine, tangent, cotangent, secant, and cosecant. An important thing to note is that the reciprocal of sine is not the inverse function of sine, that is, the cosecant function is not the inverse function of sine. So, we have cosecant which is the reciprocal of sine. In this article, let us learn about the properties of the reciprocal of sine, that is, cosecant, its formula, domain, range , derivative, integral and graph. The reciprocal of the sine function is a trigonometric function , called the cosecant function. The reciprocal of the cosecant function is the sine function itself. The product of the reciprocal of sine and the sine function is always equal to 1.

Lego technic mclaren f1

Andrew M. Have questions on basic mathematical concepts? Cosecant is the reciprocal of sin that is the ratio between the hypotenuse and the opposite side. Sal finds all six trigonometric ratios sine, cosine, tangent, secant, cosecant, and cotangent of an angle in a given right triangle. And the adjacent side, we already figure out, has length 5. Article Tags :. Hence, cosecant is the reciprocal of sine. Flag Button navigates to signup page. Remember that when you figure out a value's reciprocal, you just "flip" it as a fraction; the numerator becomes the denominator and vice versa. This article is being improved by another user right now. Terms and Conditions.

The three basic trigonometric functions occur so often as the denominator of a fraction that it is convenient to give names to their reciprocals. We define three new trigonometric functions as follows. We can find exact values for all six trig functions at a given angle if we know the value of any one of them.

The hypotenuse would be the same regardless of what angle you pick, but the opposite and the adjacent is dependent on the angle that we choose in the right triangle. The reciprocal of sine is the cosecant function. And this is all specific to angle A. In other words, the reciprocal of sine is the ratio of the hypotenuse to the perpendicular in a right-angled triangle. You can suggest the changes for now and it will be under the article's discussion tab. Posted 8 years ago. It's the longest side of the right triangle. The reciprocal trigonometric ratios. Sine is the ratio of the opposite side to the Hypotenuse. And the adjacent side, we already figure out, has length 5. Graph of Reciprocal of Sine 5.