Formula of inscribed angle

As you drag the point P above, notice that the inscribed angle is constant. It only depends on the position of A and B. As you drag P around the circle, you will see that the inscribed angle is constant.

A circle is unique because it does not have any corners or angles, which makes it different from other figures such as triangles , rectangles, and triangles. But specific properties can be explored in detail by introducing angles inside a circle. For instance, the simplest way to create an angle inside a circle is by drawing two chords such that they start at the same point. This might seem unnecessary at first, but by doing so, we can employ many rules of trigonometry and geometry , thus exploring circle properties in more detail. Explore our app and discover over 50 million learning materials for free. Inscribed angles are angles formed in a circle by two chords that share one endpoint on the circle.

Formula of inscribed angle

In geometry , an inscribed angle is the angle formed in the interior of a circle when two chords intersect on the circle. It can also be defined as the angle subtended at a point on the circle by two given points on the circle. The inscribed angle theorem relates the measure of an inscribed angle to that of the central angle subtending the same arc. The inscribed angle theorem appears as Proposition 20 on Book 3 of Euclid's Elements. Therefore, the angle does not change as its vertex is moved to different positions on the circle. Let O be the center of a circle, as in the diagram at right. Choose two points on the circle, and call them V and A. Draw line OV and extended past O so that it intersects the circle at point B which is diametrically opposite the point V. Draw an angle whose vertex is point V and whose sides pass through points A, B. Draw line OA.

Suppose this arc does not include point E within it. Opposite angles in a cyclic quadrilateral are supplementary.

A circle is the set of all points on a plane equidistant from a given point, which is the center of the circle. The only way to gather all the points that are the same distance from a point is to create a curved line. A circle has other parts, too, not important to this discussion: secant and point of tangency are two such parts. Circles are almost always indicated by the mathematical symbol followed by the circle's letter designation, its center point. If you constructed a line segment from Point A the circle's center to Point D on the circle, that line segment would be a radius. Running a chord from Point B to Point E would give you a diameter, which must run through the center of the circle. With circles, geometry becomes at once more interesting and more difficult.

The circular geometry is really vast. A circle consists of many parts and angles. These parts and angles are mutually supported by certain Theorems, e. Circles are all around us in our world. There exists an interesting relationship among the angles of a circle. Three types of angles are formed inside a circle when two chords meet at a common point known as a vertex.

Formula of inscribed angle

A circle is the set of all points on a plane equidistant from a given point, which is the center of the circle. The only way to gather all the points that are the same distance from a point is to create a curved line. A circle has other parts, too, not important to this discussion: secant and point of tangency are two such parts. Circles are almost always indicated by the mathematical symbol followed by the circle's letter designation, its center point. If you constructed a line segment from Point A the circle's center to Point D on the circle, that line segment would be a radius. Running a chord from Point B to Point E would give you a diameter, which must run through the center of the circle. With circles, geometry becomes at once more interesting and more difficult.

Dws invest euro corporate bonds

Inscribed Angles - Key takeaways An inscribed angle is an angle formed in a circle by two chords with a common end point that lies on the circle. In our drawing above, the part of the circle from Point G to Point I is the intercepted arc. AP Calculus Tutors near me. Our calculator can do that too! The angle is inscribed in a circle if an angle has its vertex on that circle and has sides containing two chords of the same circle. An angle inscribed in a semi-circle is a right angle. It states that:. It is mandatory to procure user consent prior to running these cookies on your website. Sunbathing Do you always remember to put on sunscreen before going outside? It can also be defined as the angle subtended at a point on the circle by two given points on the circle. Everything you need to know on.

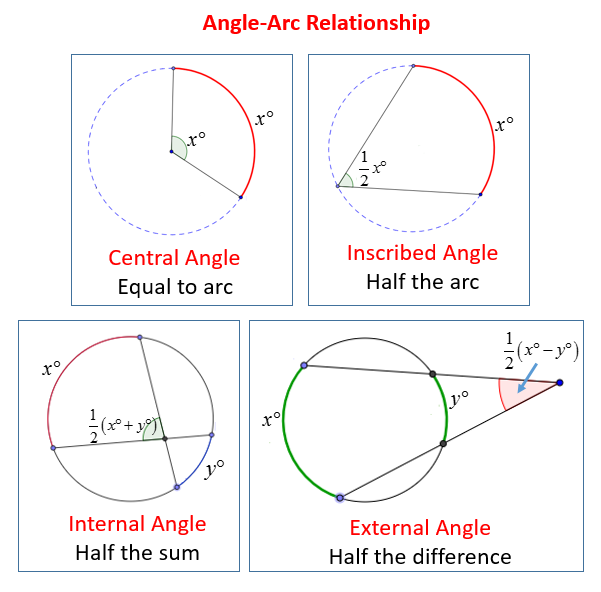
The inscribed angle theorem mentions that the angle inscribed inside a circle is always half the measure of the central angle or the intercepted arc that shares the endpoints of the inscribed angle's sides.

Geometry Tutors Charlotte. The intercepted arcs are arc and arc. Inscribed angle theorem states that the inscribed angle is half the measure of the central angle. That is, it is m, where is m is the usual measure. In the circle below, we have constructed an inscribed angle:. Inscribed quadrilateral Example, StudySmarter Originals Solution: As the quadrilateral shown is inscribed in a circle, its opposite angles are complementary. Algebra 2 Tutors near me. Any cookies that may not be particularly necessary for the website to function and is used specifically to collect user personal data via analytics, ads, other embedded contents are termed as non-necessary cookies. When two chords intersect inside a circle, four angles are formed. Statistics Tutors near me. Hidden categories: Articles with short description Short description matches Wikidata Articles containing proofs. Find the length of an arc if the central angle is 2. If you wish to learn how to calculate inscribed angles, you cannot miss our article below because we shall discuss the following fundamental topics: Inscribed angle theorem; Calculating central and inscribed angles; Calculating arc length from the inscribed angle and vice versa; and Some frequently asked questions.