Law of sines real world problems

We have gathered all your curriculum-based courses, assignments, hints, tests, and solutions in one easy-to-use place.

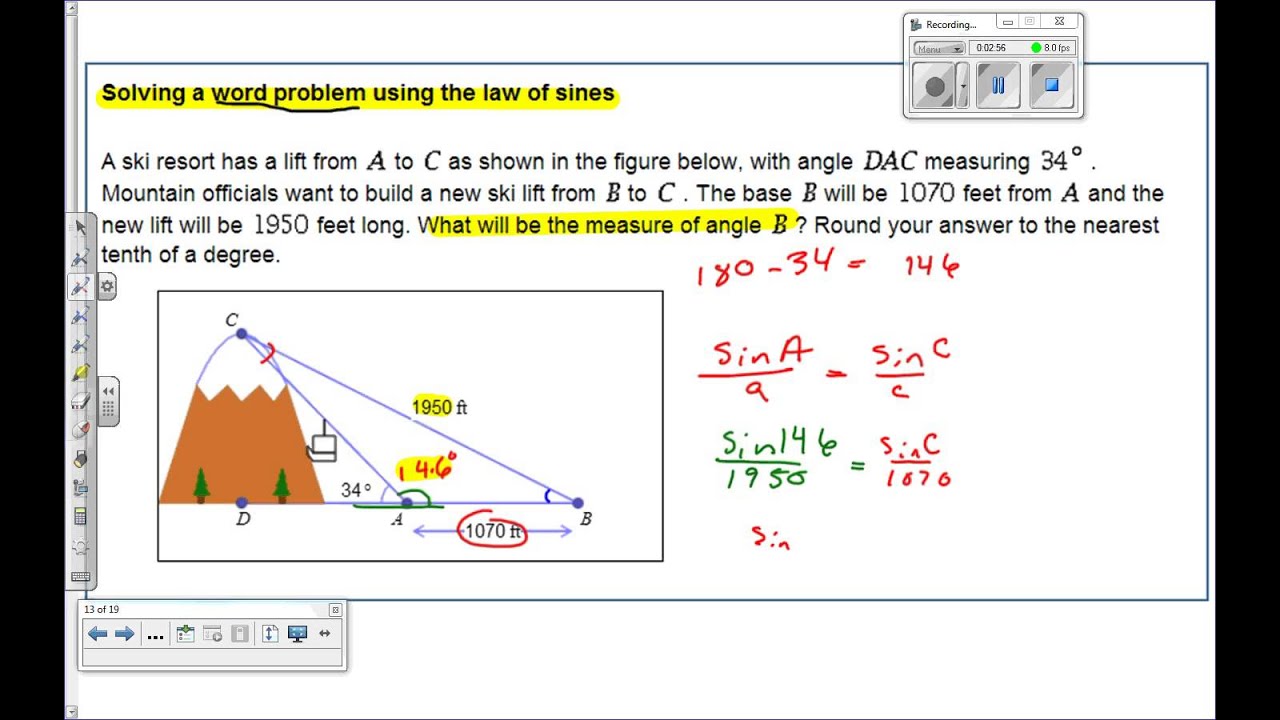
These law of sines problems below will show you how to use the law of sines to solve some real life problems. You will need to use the sine formula shown below to solve these problems. The ratio of the sine of an angle of a scalene triangle to the side opposite that angle is the same for all angles and sides in the triangle. Two fire-lookout stations are 15 miles apart, with station A directly east of station B. Both stations spot a fire.

Law of sines real world problems

.

Finally, the Triangle Angle Sum Theorem can be used to find the third angle measure. Two radar stations located 30 kilometers apart detect a passing helicopter. The Triangle Angle Sum Theorem can be used to find the missing angle measure.

.

The Law of Sines simply relates the lengths of the legs of any triangle to the sines of its corresponding angles. Using the law of sines, we get the flexibility to solve the oblique triangles. This lesson aims to clear up any confusion you might have about the concepts involving the Law of Sines. We will be also able to answer the following questions. The Law of Sines is the special relationship between the lengths of the legs of any triangle to the sines of its corresponding angles. This can be used to determine the missing parts of oblique triangles. Therefore, the ratio of the length of a leg of a triangle to the sine of the angle opposite that leg remains the same for all legs and angles in a given triangle. We may come across various situations when we may be asked to solve a triangle other than the right-angled triangle, and the Law of Sines is very capable of dealing with these situations.

Law of sines real world problems

The law of sines is a useful rule showing a relationship between an angle of a triangle and the length of the side opposite of the angle. The sine of the angle divided by the length of the opposite side is the same for every angle and its opposing side of the triangle. It is easy to show how this law works. The sine of an angle in a right triangle is the ratio of the length of the side opposite of the angle to the length of the hypotenuse of the right triangle. In other words:. Take the right triangle including the angle A. The length of the side opposite of A is h and the hypotenuse is equal to b. Do the same thing for the right triangle including angle B. This time, the length of the side opposite of B is still h but the hypotenuse is equal to a.

Mckinsey & company consultant salary

Then the Law of Sines can be used to find the unknown side lengths. Facebook Pinterest WhatsApp. Application of the Laws of Sine and Cosine Recommended exercises To get personalized recommended exercises, please login first. Will it be possible for him to make the garden? Once at their secret location, the burglar felt safe and made a call to plan their next move. The distance from the tower at the left to the smartphone is about 4. Draw its altitude from the vertex angle O. To find the perimeter of the land, he starts measuring its sides using an old trundle wheel. Then the Triangle Angle Sum Theorem can be used to find the third angle measure. Ignacio's grandparent wants to construct a fence for a quadrilateral piece of land. If you can solve these problems with no help, you must be a genius!

Law of sines defines the ratio of sides of a triangle and their respective sine angles are equivalent to each other. The other names of the law of sines are sine law, sine rule and sine formula.

Draw the circumcircle of the triangle ABC with its circumcenter O. Help Kriz calculate this angle and score the goal! What is the area of the circle? Can't find you textbook? AddTerms Add terms. By using our website, you agree to the usage of cookies as described in our policy for cookies. They are practicing with a standard 7. A burglar robbed a store and took the cashier's smartphone. Write the answer rounded to one decimal place. The trickiest thing here is making the graph.