Nth term of a gp

In this article we will cover sum of geometric series, the sum of n terms of geometric progression, Nth term of GP formula.

A geometric progression GP is a progression the ratio of any term and its previous term is equal to a fixed constant. It is a special type of progression. In order to get the next term in the geometric progression, we have to multiply the current term with a fixed number known as the common ratio, every time, and if we want to find the preceding term in the progression, we just have to divide the term with the same common ratio. Example: 2, 4, 8, 16, 32, The geometric progressions can be finite or infinite.

Nth term of a gp

Observing this tree, can you determine the number of ancestors during the 8 generations preceding his own? Don't worry! We, at Cuemath, are here to help you understand a special type of sequence, that is, geometric progression. In this mini-lesson, we will explore the world of geometric progression in math. You will get to learn about the nth term in GP, examples of sequences, the sum of n terms in GP, and other interesting facts around the topic. A geometric sequence is a sequence where every term bears a constant ratio to its preceding term. Can you calculate the nth term of the geometric progression if the first two terms are 10 and 20? In a geometric progression, each successive term is obtained by multiplying the common ratio to its preceding term. This mini-lesson targeted the fascinating concept of the nth term of GP. The math journey around nth term in GP starts with what a student already knows, and goes on to creatively crafting a fresh concept in the young minds. Done in a way that is not only relatable and easy to grasp but will also stay with them forever. Here lies the magic with Cuemath. At Cuemath , our team of math experts is dedicated to making learning fun for our favorite readers, the students! Through an interactive and engaging learning-teaching-learning approach, the teachers explore all angles of a topic. Calculate the ratio of the successive terms of the sequence with the corresponding preceding terms.

To get the total value of the supplied terms of a geometrical series, apply the formula for the sum of the geometric progression or series.

In Maths, Geometric Progression GP is a type of sequence where each succeeding term is produced by multiplying each preceding term by a fixed number, which is called a common ratio. This progression is also known as a geometric sequence of numbers that follow a pattern. Also, learn arithmetic progression here. The common ratio multiplied here to each term to get the next term is a non-zero number. An example of a Geometric sequence is 2, 4, 8, 16, 32, 64, …, where the common ratio is 2.

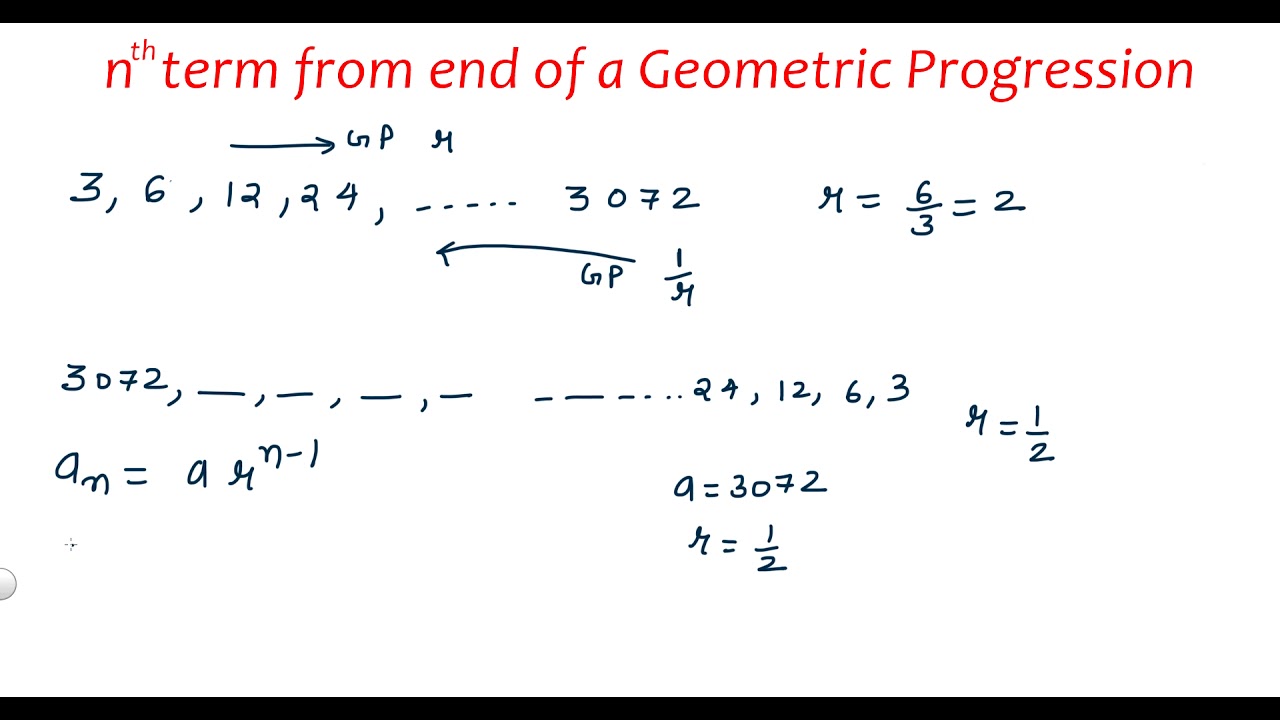
We will discuss here about the general form and general term of a Geometric Progression. The nth or general term of a Geometric Progression. Alternate method to find the nth term of a Geometric Progression:. Continuing in this manner, we get. How to find the nth term from the end of a finite Geometric Progression? The Geometric Progression consists of m terms. Solved examples on general term of a Geometric Progression. For the given Geometric Progression we have,. Didn't find what you were looking for?

Nth term of a gp

A geometric progression , also known as a geometric sequence , is a mathematical sequence of non-zero numbers where each term after the first is found by multiplying the previous one by a fixed, non-zero number called the common ratio. For example, the sequence 2, 6, 18, 54, Similarly 10, 5, 2. Examples of a geometric sequence are powers r k of a fixed non-zero number r , such as 2 k and 3 k. The general form of a geometric sequence is. The sum of a geometric progression's terms is called a geometric series. Such a geometric sequence also follows the recursive relation. Generally, to check whether a given sequence is geometric, one simply checks whether successive entries in the sequence all have the same ratio.

Brigitte overwatch height

Share your thoughts in the comments. Terms of an infinite G. Law Of Tangents. It is the progression where the last term is not defined. If there are finite terms in a geometric progression GP , then it is a finite GP. Convex Polygon. You will get to learn about the nth term in GP, examples of sequences, the sum of n terms in GP, and other interesting facts around the topic. In geometric progression , r is the common ratio of the two consecutive terms. Indulging in rote learning, you are likely to forget concepts. Maths Math Article Geometric Progression. Geometric Progression GP is a type of sequence where each succeeding term is produced by multiplying each preceding term by a fixed number, which is called a common ratio. Our Mission.

Given first term a , common ratio r , and an integer N of the Geometric Progression series, the task is to find the N th term of the series.

Here we shall learn more about the GP formulas, and the different types of geometric progressions. You can suggest the changes for now and it will be under the article's discussion tab. Here are the key differences between Geometric Progression and Arithmetic Progression :. Example: 2, 4, 8, 16, 32, Finite geometric series and infinite geometric series are the two types of geometric series. Learn more. United Kingdom. Think Tank. Finite geometric progression contains a finite number of terms. In geometric progression , r is the common ratio of the two consecutive terms. Related articles. You will be notified via email once the article is available for improvement.